


Next: About this document
Study Guide for Final Examination
Massachusetts Institute of Technology
Department of Physics
Physics 8.04 May 18, 1997
The material which the final examination will cover includes the
material covered on the first and second quizzes as well as bound
state solutions for the Dirac  -function and the one
dimensional simple harmonic oscillator, and scattering problems in one
dimension including computing reflection and transmission
probabilities and packet delay times for sharp potential steps,
resonant transmission across a barrier, tunneling through a barrier,
and resonant tunneling through a double barrier, and scattering
problems involving
-function and the one
dimensional simple harmonic oscillator, and scattering problems in one
dimension including computing reflection and transmission
probabilities and packet delay times for sharp potential steps,
resonant transmission across a barrier, tunneling through a barrier,
and resonant tunneling through a double barrier, and scattering
problems involving  -potentials.
-potentials.
The material covered on the final includes but need not be limited to
the material in the outline below.
Quantum Theory: a fundamental description of the behavior of matter
and energy
- Experiments establishing the identity/nature of the building
blocks of the microscopic world
- Nuclei -- Rutherford Scattering
- Electrons (
 )
)
- J.J. Thomson's,
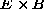 experiment
experiment 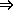 measure e/m
measure e/m - R.A. Millikan's oil drop experiment
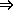 measure e
measure e - Davisson-Germer/G.P. Thomson Experiments
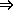 wave-like nature of electrons with
wave-like nature of electrons with 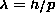
- Classical mechanics (
 )
+ correspondence principle (
)
+ correspondence principle ( 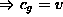 ) leads to the De
Broglie Hypotheses,
) leads to the De
Broglie Hypotheses, 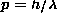 and
and 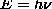 . From this
argument we see why both the energy-frequency and the wavelength-momentum
h's are the same and why the h's are the same for all objects.
. From this
argument we see why both the energy-frequency and the wavelength-momentum
h's are the same and why the h's are the same for all objects. - Double-slit thought-experiments (
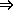 interference patterns) + the discreteness of microscopic events leads
to the idea that microscopic events are probabilistic in nature but
with a predictable distribution. We find that the probability
of an event
interference patterns) + the discreteness of microscopic events leads
to the idea that microscopic events are probabilistic in nature but
with a predictable distribution. We find that the probability
of an event 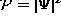 is the complex magnitude squared of a
wave-like amplitude. The amplitude function
is the complex magnitude squared of a
wave-like amplitude. The amplitude function 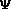 somehow senses and
propagates through the entire system including any apparatus
making observations of the system.
somehow senses and
propagates through the entire system including any apparatus
making observations of the system. - Single-slit thought-experiments lead to the Heisenberg
Uncertainty Principle [HUP] as a basic property of matter:
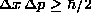 .
Applications of HUP:
.
Applications of HUP:
- Limits on the predictability of classical trajectories: quantum pin-ball
- Ground state energies:
Particle in a box:
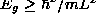 (energy of confinement)
Simple harmonic oscillator [SHO]:
(energy of confinement)
Simple harmonic oscillator [SHO]: 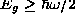 Hydrogen Atom [H-atom]:
Hydrogen Atom [H-atom]: 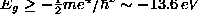 (explains stability, size and energy of atoms)
(explains stability, size and energy of atoms)
- Photons
- A. Einstein, photoelectric effect
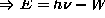
- Inverse photoelectric effect Bremsstrahlung/X-ray-emission
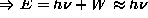
- GI Taylor: single-photon interference experiments showing
the probabilistic nature of the arrival of individual photons.
- Classical E&M experiments (Maxwell's equations
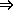 E=cp) + Correspondence
Principle (
E=cp) + Correspondence
Principle ( 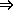 large numbers of photons en masse act like classical
E&M) and Planck's relation (
large numbers of photons en masse act like classical
E&M) and Planck's relation ( 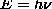 ) leads to the
conjecture that
) leads to the
conjecture that 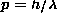 for photons as well as for particles
for photons as well as for particles
- Putting the building blocks together
- Compton Scattering
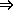 confirms
confirms 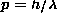 experimentally
experimentally - Atomic Spectra: Balmer Series, etc.
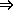
 with
with 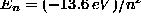 .
. 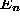 may be understood
with Bohr's quantization condition
may be understood
with Bohr's quantization condition 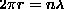 . To get
perfect alignment with the
experiments must use the effective mass
. To get
perfect alignment with the
experiments must use the effective mass 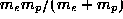 !
!
- Franck-Hertz
 verification of existence of
discrete internal energy states
verification of existence of
discrete internal energy states - Mosely's X-ray spectra of multi-electron atoms
 basic concepts work if a shell-structure is assumed for the electrons
basic concepts work if a shell-structure is assumed for the electrons - Closer Look at Semiclassical Quantization
- Quantization condition:
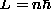 ,
, 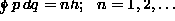
- Examples:
- Particle in a box:
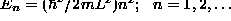
- Simple Harmonic Oscillator [SHO]:
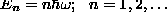
- Hydrogen Atom [H-atom]:
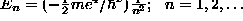
- Correspondence Principle/Classical Limit
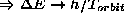
- de Broglie Hypothesis:
An electron or photon represents the possibility for the occurrence of
a discrete microscopic event, where the probability of that
event may be described by a superposition of waves with
frequency 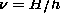 and wavelength
and wavelength
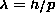 , where H is the Hamiltonian, p is the
momentum for the electron or photon, and h is Planck's constant
, where H is the Hamiltonian, p is the
momentum for the electron or photon, and h is Planck's constant
- Quantum States and Observables
- Quantum State -- two systems are defined as being in the same quantum state if
and only if the statistical results of all experiments carried out on
those systems are identical.
- Pure Quantum State -- a system is in a pure quantum state
with respect to an observable if all measurements of that
observable always yield the same value.
- Specification of Quantum States -- specifying the probability
distribution for just one observable is, in general, insufficient to
specify the quantum state of a system.
- Representation of Quantum States -- the set of
quantum amplitudes (either
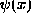 for all x,
for all x, 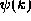 for all k, or
for all k, or 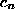 for all n) in a particular representation
(position, momentum, energy) are sufficient to specify a quantum
state. Students should be able to transform readily between different
representations.
for all n) in a particular representation
(position, momentum, energy) are sufficient to specify a quantum
state. Students should be able to transform readily between different
representations.
- Distributions, Averages and Operators
- The probability of measuring a particular value of a
particular observable is given by the complex magnitude squared of the
quantum amplitude in that representation.
 ,
,
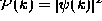 ,
, 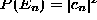 .
. - Averages may be computed directly from the probability
distributions (e.g.,
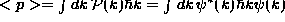 ) or through use of the appropriate operators
) or through use of the appropriate operators
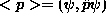 .
. - Students should be comfortable with the use of the Hermitian
inner product
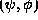 and basic operator operations such as
and basic operator operations such as
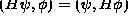 or
or 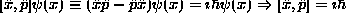 .
. - Students should be able to apply the ``Quantum Purity Test'' to
determine whether a quantum state is pure with respect to a particular observable by applying the corresponding operator:
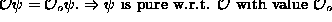 .
.
- Time Dependent Schrödinger Equation (TDSE)
- Students should be familiar with the origins and form of the
TDSE.
- Students should be familiar with and able to apply the
conservation of probability, the continuity equation and the
probability current.
- Students should be familiar with and able to apply
Ehrenfest's theorem, both in the case of
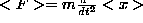 and in its general form
and in its general form 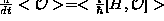 for any operator
for any operator 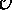 .
. - Students should be familiar with and able to apply the formal
solution to the TDSE:
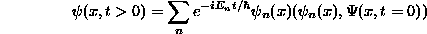
- Time Independent Schrödinger Equation (TISE)
- Students should recognize the TISE as the quantum purity test for
pure states of energy.
- Students should be familiar with the expansion
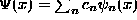 and be able to determine the constants
and be able to determine the constants
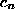 for any given function
for any given function 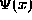 through the relation
through the relation
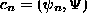 .
. - Students should be familiar with orthonormality among
eigenstates
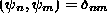 .
. - Students should be able to complete proofs using the expansions,
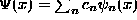 and
and 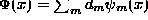 .
. - Given a wavefunction expanded at t=0,
 , students should be able to give the solution for all later
times
, students should be able to give the solution for all later
times 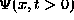 .
. - Students should be able to give qualitative sketches of bound
state solutions to the TISE.
- Applications of the TDSE
- Students should be able to use the TDSE and the Method of
Stationary Phase to describe the behavior of wave packets in
scattering problems, including being able to locate the positions of
the source, reflected and transmitted packets in terms of a) the
phases of the linear combination coefficients making up the wave
packet and b) the phases of the quantum amplitudes for the source,
reflected and transmitted beams.
- Applications of the TISE
- Students should be able to solve analytically for the bound
eigenenergies and eigenstates of the TISE in one dimensional problems
involving piecewise-constant potentials and Dirac
 -functions.
-functions. - Students should be able to reproduce each step of the solution
for the simple harmonic oscillator. This includes:
- The conversion to a dimensionless equation without the
constants
 , m, and
, m, and 
- Knowing the form of the solution as as a
product of polynomials times a Gaussian which is its own
Fourier transform:
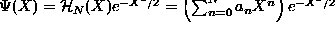
- The generation of the equation for the polynomials:
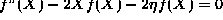
- The solution of the above equation using the power series
expansion:
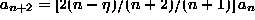
- Reconstructing the eigenstates and applying the solutions as on the problem sets
- Students should also be familiar with generating sucessive
excited states using the creation operator
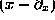 , and
also the fact that
, and
also the fact that 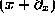 is the annihilation operator, which
produces the next lower state when applied to a pure state of the SHO.
is the annihilation operator, which
produces the next lower state when applied to a pure state of the SHO.
- Students should be able to solve analytically for the
scattering eigenstates of the TISE in one-dimensional problems
involving piecewise-constant potentials and Dirac
 -functions.
They should be able to interpret the results and from them to extract
the quantum amplitudes of transmission t(k) and reflection r(k).
From these amplitudes, students should be able to compute reflection
and transmission probabilities (
-functions.
They should be able to interpret the results and from them to extract
the quantum amplitudes of transmission t(k) and reflection r(k).
From these amplitudes, students should be able to compute reflection
and transmission probabilities ( 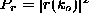 and
and  ,
respectively) and delay times (
,
respectively) and delay times (  and
and 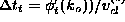 , where
, where 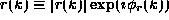 ,
, 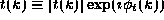 , and
, and
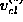 is the classical velocity in the region which is the
source of the scattered particles.
is the classical velocity in the region which is the
source of the scattered particles. - Students should be familiar with the following scattering phenomena:
- Reflection from a sudden change potential:
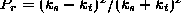
- Barrier penetration: the probability is non-zero in a
forbidden region but decays exponential with a decay constant
 . For such situations there
is a time delay in the generation of the reflection of
. For such situations there
is a time delay in the generation of the reflection of  , as though the particles first travel a
distance
, as though the particles first travel a
distance 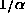 into the barrier and then the same distance
back out.
into the barrier and then the same distance
back out. - Resonant scattering across a potential well: sometimes
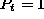 for special values of k
for special values of k - Resonant tunneling: sometimes
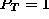 even when tunneling is
involved so long as there is a resonance region separated by two
symmetric, opposing scatterers
even when tunneling is
involved so long as there is a resonance region separated by two
symmetric, opposing scatterers
- Feynman diagrams: Students should be able to perform Feynman
sums over histories to solve scattering problems. This includes



Next: About this document
Prof. Tomas Alberto Arias
Sun May 18 10:23:16 EDT 1997
![]() -function and the one
dimensional simple harmonic oscillator, and scattering problems in one
dimension including computing reflection and transmission
probabilities and packet delay times for sharp potential steps,
resonant transmission across a barrier, tunneling through a barrier,
and resonant tunneling through a double barrier, and scattering
problems involving
-function and the one
dimensional simple harmonic oscillator, and scattering problems in one
dimension including computing reflection and transmission
probabilities and packet delay times for sharp potential steps,
resonant transmission across a barrier, tunneling through a barrier,
and resonant tunneling through a double barrier, and scattering
problems involving ![]() -potentials.
-potentials.
![]() and wavelength
and wavelength
![]() , where H is the Hamiltonian, p is the
momentum for the electron or photon, and h is Planck's constant
, where H is the Hamiltonian, p is the
momentum for the electron or photon, and h is Planck's constant![]()