



Next: About this document
Up: What does it all
Previous: Fixed/closed boundary condition:
(Eq. )
24
If an end of the string at location
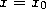 is completely free to move in the y-direction, then the
solution must approach that point with zero slope at all times t.
Physically, we can understand this because we can imagine the free end
of the string to be tied to a massless ring tied to a
frictionless rod. Because the ring is massless and because of
Newton's law F=m a, there cannot be any force on the ring in the
y-direction. Any slope in the string, however, would immediately
create a tension force on the ring in the y-direction according to
our result for the vertical components of the tension. Thus, the
solution y(x,t) must always approach a free-end with zero slope.
is completely free to move in the y-direction, then the
solution must approach that point with zero slope at all times t.
Physically, we can understand this because we can imagine the free end
of the string to be tied to a massless ring tied to a
frictionless rod. Because the ring is massless and because of
Newton's law F=m a, there cannot be any force on the ring in the
y-direction. Any slope in the string, however, would immediately
create a tension force on the ring in the y-direction according to
our result for the vertical components of the tension. Thus, the
solution y(x,t) must always approach a free-end with zero slope.
Tomas Arias
Mon Oct 15 16:15:07 EDT 2001