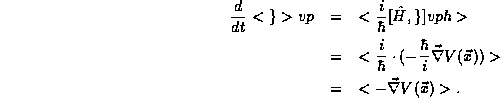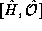 using the
specific forms of the operator
using the
specific forms of the operator  , and the operators
, and the operators 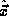 and
and 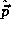 . We will begin with the position operator
. We will begin with the position operator 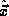 ,
,
To apply our general result (12) to prove Ehrenfest's
theorem, we must now compute the commutator 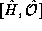 using the
specific forms of the operator
using the
specific forms of the operator  , and the operators
, and the operators 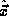 and
and 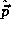 . We will begin with the position operator
. We will begin with the position operator 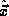 ,
,

Inserting this into (12) completes the proof of the first part of Ehrenfest's Theorem,

The proof of the second part of Ehrenfest's theorem proceeds in the
precisely the same way, but now we must compute the commutator
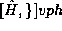 ,
,
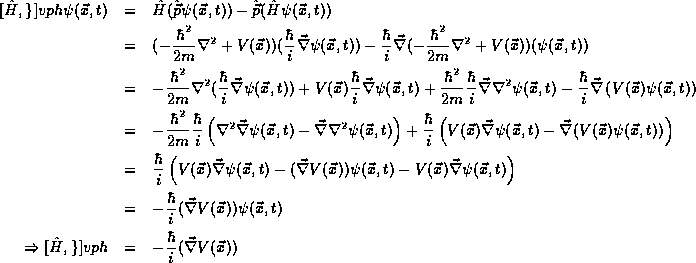
Inserting this into (12) completes the proof of second part of Ehrenfest's Theorem,