Equation of motion --

The equation of motion expresses the controlling physical laws in terms of nothing other than the degrees of freedom, derivatives of the degrees of freedom and given physical quantities and constants. As for the physical laws, the problem involves various types of energy, whose definitions we must invoke, and also involves the quantum mechanical behavior of particles, which the de Broglie hypothesis describes. Thus, we can express the basic physical laws as

$$E \equiv \frac{p(x)^2}{2m} + U(x) \mbox{\ \ \ ; Definitions of energy}$$

$$= \frac{\left( \hbar k(x) \right)^2}{2m} + U(x), \mbox{\ \ \ ; de Broglie hypothesis}$$ (16)

where we have been cautious to note that the presence of the force means that the particle will have different velocities and thus different momenta 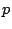$p$ and wave vectors 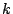$k$ at each point $x$. This equation expresses all of the physical laws in a single equation; however, it uses $k(x)$ which, unlike all other quantities in the equation, is not a given quantity. To complete the equation of motion, we must find a way to express the wave vector directly in terms of the degrees of freedom $\Psi(x)$ at its derivatives.

To find such an expression, first recall that the wave vector 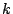$k$ counts up how many oscillations there are in $\Psi(x)$ per unit length. This kind of counting is difficult to express in terms of the value of $\Psi(x)$ and its derivatives. However, the number of oscillations per unit length is certainly related to the curvature of $\Psi(x)$ - the more curvature, the more oscillations per unit length. To see this mathematically, consider the second derivative (which gives the curvature) of any pure sinusoidal wave of arbitrary amplitude and phase,

$$\begin{eqnarray*} \Psi(x) & = & A \cos(kx + \phi_0) \\ \Psi'(x) & = & -k A \sin... ..._0) \\ \Psi''(x) & = & -k^2 A \cos(kx + \phi_0) = -k^2 \Psi(x). \end{eqnarray*}$$

Thus, we see that we can get a measure of the wave vector 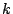$k$ at any point by looking at the ratio of the second derivative to the value of the function,

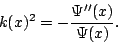$$k(x)^2 = - \frac{\Psi''(x)}{\Psi(x)}.$$ (17)

Substituting the result Eq. (17) into Eq. (16) gives the final equation of motion,

$$E = -\frac{\hbar^2}{2m} \frac{\Psi''(x)}{\Psi(x)}+U(x).$$

Or, equivalently,

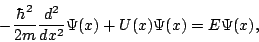$$-\frac{\hbar^2}{2m} \frac{d^2}{dx^2} \Psi(x) + U(x) \Psi(x) = E \Psi(x),$$ (18)

where in the last line we have rearranged things somewhat to correspond to the standard way of writing the quantum equation of motion, the famous Schrödinger Equation.