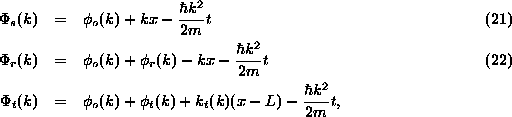
The phases of the three packets 17-19 are
respectively. Evaluating the corresponding stationary phase conditions gives
for the location of the source packet,
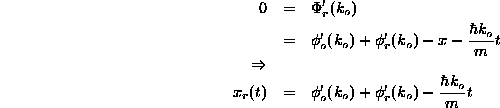
for the location of the reflected packet, and
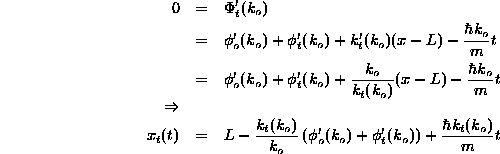
for the transmitted packet. For evaluation of the expression for the transmitted packet, we have used the identity,
where as in Section
3.3, 
.
We first note that in all three cases, we find packets traveling with
the expected classical velocities, 
,
and 
, for
the source, reflected and transmitted packets, respectively.
Let us call t=0 a time when the source packet 
is
entirely within Region s. The source packet then begins at 
and then propagates to the right, until at time
, its center reaches the point x=0
where the particle first ``collides'' with the collision region. (See
Figure 8.) At this moment, half of the packet extends into
Region c. Note that because is only evaluated in
Region s in (20), the part of the source packet
extending into Region c makes no contribution to the final wave
function 
. As time goes on, eventually
enters Regions c and t entirely, at which point the source packet
entirely disappears from the problem.
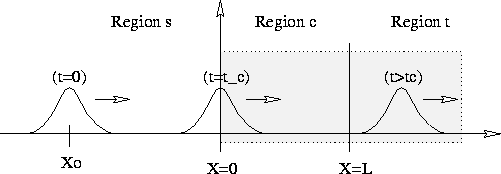
Figure 8: Evolution of the source packet in a general scattering problem
The location of the reflected packet at time t=0 is
, which then must be entirely within
Regions c and t. At this point in time, the reflected packet makes no contribution to the
problem. ( 
is only evaluated in Region s in
(20).) As this packet then travels to the left,
eventually, at time 
, it reaches the point x=0
and emerges into Region s, from where it continues back toward the
source. (See Figure 9.) Note that the time 
at which
the reflection emerges into the problem may is delayed by a period
from the moment when the source packet first collides with the scattering region.
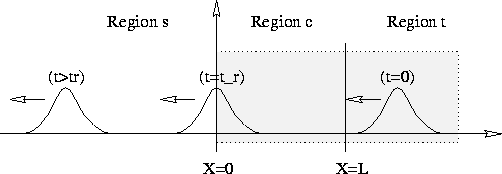
Figure 9: Evolution of the reflected packet in a general scattering problem
At t=0, the transmitted packet 
is located at
and contained
within within Regions s and c. This packet eventually emerges into the
problem at the moment when 
, at time
which is a period
later than the moment 
when the source packet collides with the
scattering region. (See Figure 10.)
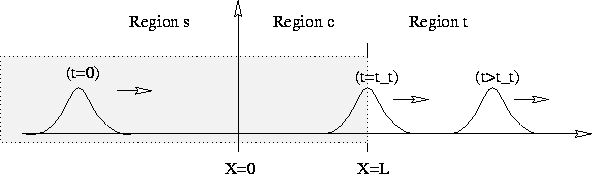
Figure 10: Evolution of the transmitted packet in a general scattering problem
Taken all together, describes the following sequence of
events. First, there is only a packet from the source traveling to
the right in Region s at velocity 
. Then, at time
determined by the initial conditions,
this packet collides with the scattering region, Region c. A period
later, which is characteristic
of the scattering potential only, a reflected packet emerges back from
Region c into Region s and then continues to move to the left with
velocity 
. An additional transmitted packet emerges
into Region t a period 
, which again is a
characteristic of the scattering potential only, after the collision
at time 
and travels to the right with velocity 
.
The order in which the reflected and transmitted packets emerge
depends on the sizes of 
and 
.
