We have found that the quite disparate systems of a string,
pressure wave (in a gas, fluid or solid), and vacuum (or a material
with internal electric and magnetic fields) all ultimately obey the
same wave equation, 
, where q is some quantity describing the degrees of
freedom in the system.
We have found there to be deeper connections, however, which help to explain the ubiquity of the wave equation. On the string and in sound, we can define a quantity describing driving forces in the system in terms of the first spatial derivative of the solution and a quantity describing momentum in the system in terms of the first time derivative of the solution. We then find that the wave equation comes from relating the first spatial derivative of the driving forces (which gives the net force on a chunk) to the first time derivative of the momentum density, which is just Newton's law that total net force equals rate of change of momentum. For electromagnetics, there is no longer Newton's law but Maxwell's equations. Nonetheless, we find exactly analogous quantities and equations. Table 1 summaries the analogy.
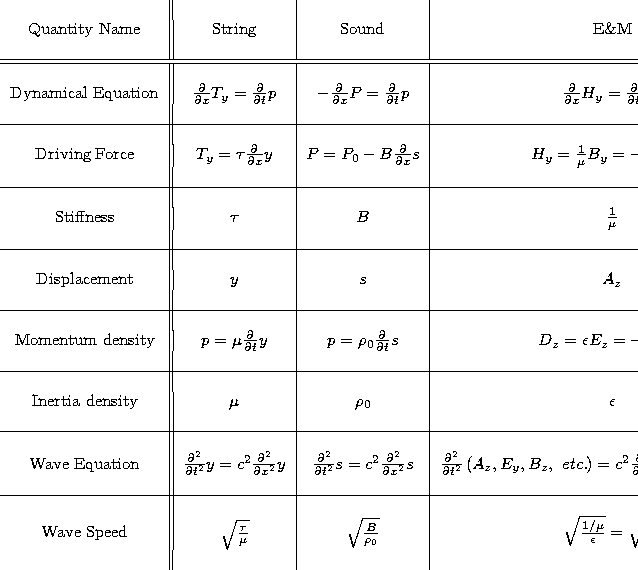
Table 1: Physics analogy among strings, sound, and E&M systems