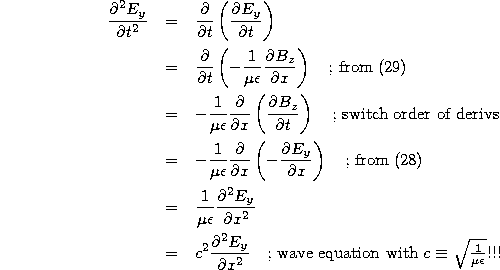
Now in possession of the laws of motion in differential form (27,28,29,30), we have only to see whether the components of the fields actually satisfy the wave equation. We begin with
Thus, indeed, the y-component of the electric field satisfies the wave equation and exhibits all the same wave phenomena (such as standing waves) as do strings and sound. Using the values of the constants appropriate for vacuum (18), we find the speed of these waves to be
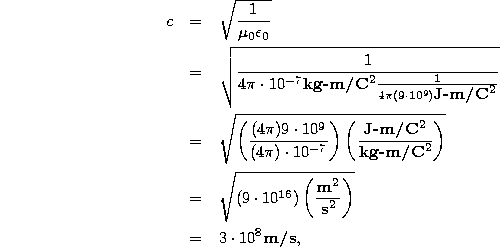
which is precisely the speed of light in vacuum!!! We can imagine
what a day Maxwell must have had when he first obtained this result.
This establishes that the light that we see actually may be understood
as electromagnetic waves. Amazingly, the constants 
and
of ordinary E&M laboratory experiments involving charges,
currents and electric and magnetic fields are precisely what are
needed to understand the seemingly completely different phenomenon of
light!
Following the same steps that we did to derive (32), we can verify that all of the other components of the fields satisfy the wave equation. The key observation here is that they all obey the same wave equation with the same wave speed, so that the fields always move together. These derivations all follow the same general strategy of starting with the second time derivative, using one of the differential versions of Maxwell's equation, switching the order of the resulting mixed partial derivatives, and then using the differential versions of another Maxwell's equation.
