We conclude with a beautifully simple derivation of a very famous
approximation due to Wentzel, Kramers and Brillouin for the form of
the electronic wave function in a very smooth potential. The basis for our
approach is that a smooth potential may be well approximated as a
series of very small steps of width 
, as Figure 22
shows. So long as we avoid regions such as b;SPMlt;x;SPMlt;c where the step
heights become comparable to the difference between the the potential
and total energies, then the reflection coefficients such as
(14) and its forbidden analogue in Section
3.6 will be very small and the stepped potential acts
like the smooth potential which it is supposed to mimic.
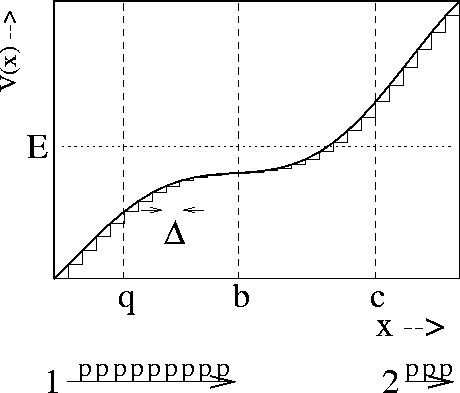
Figure 22: Smooth Potential Viewed as a series of small steps
Because the reflection amplitudes are small within these regions, we may ignore diagrams involving reflections because they give negligible contributions to the final result. The entire Feynman sum for the propagating wave function then reduces to a single diagram progressing to the right. Figure 22 gives two such examples, one in a classically allowed region and the other in a classically forbidden region.
Corresponding to the small reflection amplitudes, the transmission
amplitudes across the steps in allowed and forbidden regions are almost
exactly one. These amplitudes are the ratio of the geometric to the
algebraic mean of the wave vectors or decay constants before and
after each step, 
and
,
respectively. So long as the step size is small, 
or
, these amplitudes approach unity.
Because all of the transmission factors are nearly one, we do not even need
to list them explicitly in the amplitude for the history. The
entire Feynman sum for a smooth potential is thus approximated by a
simple product of either propagating or penetrating factors.
In the classically allowed case we then find for transmission between
two points 
and 
the following amplitude
where in the last step we have noted that in the limit of infinitely
narrow steps, the exponent just become the Riemann sum for the integral
of 
. The classically forbidden
case follows a similar logic,
where 
. The fact the
wave progresses as an integral of the local wave vector k(x) or
decay constant 
is the heart of the WKB approximation.
The WKB approximation actually goes one step further, and it is easy
for us to obtain the full expression. The usual expression for the
WKB approximation gives the value of the wave function as a function
of x. In our Feynman formulation the wave function in the region
just after the step at point q is the quantum amplitude at that
point 
at point q time the corresponding, appropriately centered
unit current,
The wave of the wave function at the point x=q is therefore
Combining this with (30) gives the value of the wave function in a smoothly varying classically allowed region as
As we discussed in Section 3.6, the unit transmitted
beam in forbidden regions is given by 
. Therefore in classically forbidden regions
we find
These last two results are the complete WKB approximation derived as just the first Feynman diagram in scattering theory!!!
