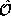The second special property of observable operators which follows from
our definition that physical operators give correct averages of
observables is Hermitianness. We say that the operator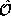 
has the
property of Hermitianness or that it is Hermitian of we may
apply the operator on either side of an inner product and always get
the same result,
Algebraically, we often wish to make this manipulation and it is good to know that it may be carried out with any observable operator. In this section we will prove that all operators associated with physical observables are Hermitian. Later you will learn that all linear, Hermitian operators are associated with physical observables.
Hermitianness of an operator is also an important concept physically.
It provides a quick test of whether an operator is physically
observable. For instance, we know that there is no physical
observable associated with the product 
because it is not
Hermitian,
The fact that the product 
is not an observable is directly
related to the Heisenberg uncertainty principle, which prevents the
simultaneous measurement of both x and p. Note also that
our analysis up to step (*) depended only on the Hermitianness of
and 
. If 
and 
were commuting operators, then
our result would have been zero for all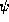 
and 
implying
that the product of two Hermitian operators is Hermitian if and only
if they commute. This example demonstrates the important fact that
although the operator product of two linear operators is always a
third linear operator, the product of two Hermitian operators
need not be Hermitian (unless they commute).
To see how Hermitianness follow from our definition of quantum observable operators, we proceed as we did with linearity. First we see that the statement is clearly true in the pure state representation of the associated observable and then we argue that the statement maintains its form in any representation. First, in the pure state representation,
Now, by Parseval's Theorem, if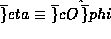 
,
then
.
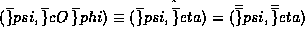By our definition of 
,
, and so
.
Similarly,
, and
thus in any representation for a physically observable operator,
The observation that physical operators are always Hermitian is a very powerful mathematical fact. While trivial in the pure state representation, Hermitianness is not always apparent in other representations. For instance, while clearly,
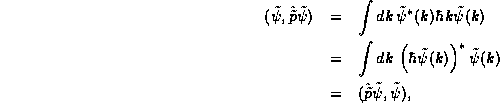
the same statement is in the position representation is much harder to prove mathematically, requiring the ``trick'' if integrating by parts at just the right place,
