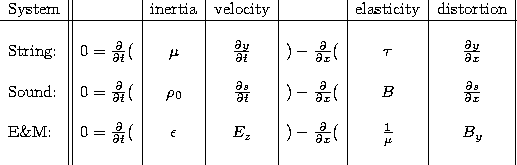
For sound and electromagnetic waves, one can carry out very similar derivations. The derivations, in fact, will be exactly the same with analogous quantities appearing in the corresponding places. Rather than repeat the same equations over and over, we choose here to show how to exploit the analogy. Following Eqs. (8) and (11) and the differential form of Ampere's law from our notes on electromagnetic waves, we have the following analogous equations for all three systems
where analogous quantities are aligned in columns.
Next, we write our result for the conservation of energy for the string at the top of a new table, and produce valid equations for the other systems by substituting the analogous quantities from the columns of (17) for each system,
All of the above equations are correct and represent the conservation
of energy for the corresponding systems. Note that, for sound and
E&M waves, which occur in three dimensions, the energy densities
ke(x) and pe(x) are per unit volume (J/m 
), and the
energy fluxes 
give power per unit area (Watt/m 
).
This later quantity actually is the physical definition of
intensity, the quantity which we studied in the previous unit in this
course. The fact that the formulas for all contain a product
of two wave variables explains why we took the ``intensity'' to be
proportional to the square of the amplitude.
A few interpretive comments are important for the E&M results. In
addition to the analogy, the table properly takes the vector nature of
the electromagnetic field into account. The analogy tell us that we
should put 
in the ``ke(x)'' column. However,
this accounts only the energy from 
. When all components are
accounted, the final result involves the total square magnitude of the
field, 
so that we put 
instead of
just 
. Similarly, for ``pe(x)'', the analogy directly tells
us to put 
, but to account for all the components
we put 
in place of 
. Finally,
the analogy would have us put 
for . Accounting
properly for all the signs involved in the equations for each of the
components, we know that the result should point in the direction of
travel of the wave, which we already learned to be along 
. Thus, the vector expression we give for is
arranged to give both the correct magnitude and direction for the
flow of energy.
As a final note, the result given for E&M is correct and represents
conservation of energy, but the terms 
and 
do not represent ``kinetic'' and ``potential'''' energy in the
usual sense. Rather, they give the energy density stored in the
electric and magnetic fields, respectively. You should recall these
energy density expressions as exactly the same expressions from your
class in electromagnetism for the energy density stored, respectively,
between the plates of a capacitor and inside of a solenoid!
